| English Page |
The unbearable lightness of coincidences |
 |
 |
| 23 October 2014 | ||||||
The Pyramids of Teotihuacan, Giza and Xianyang
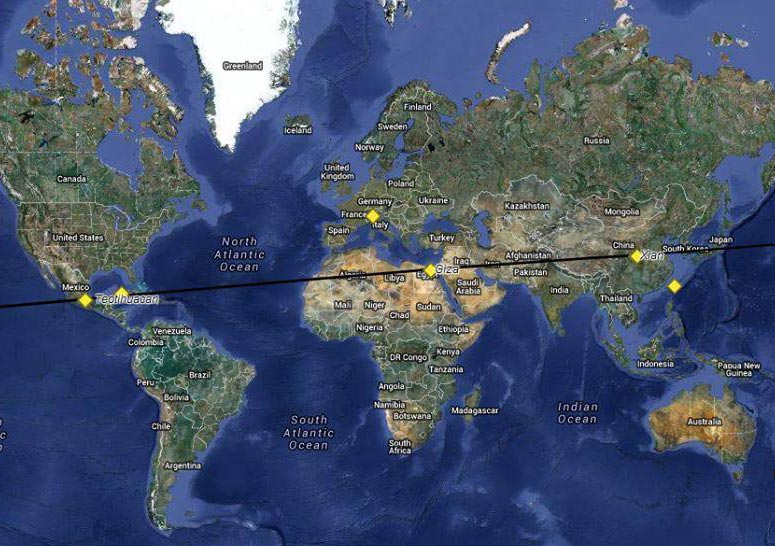
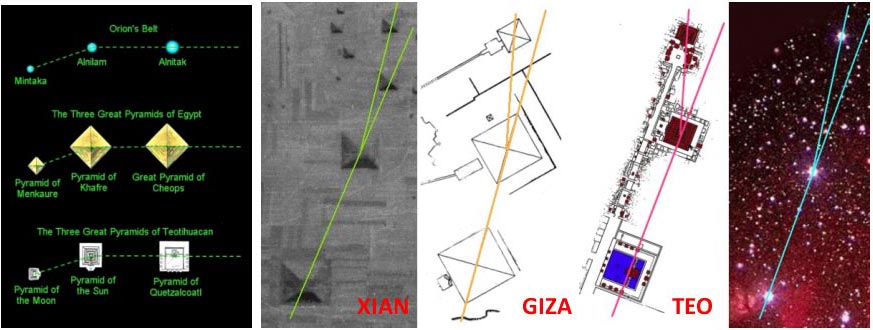
It is easy to see how the data now in our possession correspond to reality and in fact this has already been demonstrated by several experts1, as skillfully shown in these images (Image 1). If we focus in on the points of interest, we are faced with an even more special situation, of which the following picture is a representation (Image 2). In picture 1 there is no doubt of the alignment, and anyone with access to the Internet, using a program like Google Earth, can easily verify it. In picture 2, there is a greater difficulty for those without more specialised IT in making a comparison, but for the following statistical analyses, simply refer to the image 1. That said, I make 2 obvious comments, which are scientifically valid for the three most important pyramid complexes of Mexico, Egypt and China, and which are respectively located in the regions known as Teotihuacan, Giza and Xianyang: 1) They are aligned along a planetary line; 2) All of their layouts on the ground are very similar; Since these two observations have already been thoroughly investigated by scholars1 who are a lot more experienced than I, I avoid entering into the merits of the values and proofs, leaving the reader both the burden and the pleasure to explore these topics.
My aim is rather to treat the available data statistically.
We start from the observation n°1: what is the probability that 3 different people in 3 different times in 3 different continents, build 3 pyramid complexes aligning them coincidentally along one planetary line? For the convenience of readers, I will use some simple definitions: 1) Probability (classical) of an event is: the ratio between the number of favourable cases and the number of possible cases which are supposed to be equally possible 2) Coincidence (Garzanti): random circumstances coincide more often For the statistical analysis, we have to define the extent of a territory (the sample space) as a "set" of the probabilities for building sites and to identify the spatial area of the pyramid complex. The latter figure we can establish as the rectangle that contains the 3 main pyramids of each site (for brevity we will call that the "the inclusion rectangle "). In that way, we analyze the data. Unfortunately the extent of the territory used by the makers is known only at Giza,. Even in this case, however, we cannot feel that 100% of the territory of the Empire Ancient Kingdom (including the Nile, the hills, the desert, etc.) would be suitable for the construction of the pyramidal complex. To simplify the discussion, we make a conservative assumption that only 10% of the land was suitable for construction and had the right features: flat and with sufficient capacity to support the load of the pyramids. With regard to Giza, we have the following data: 1) The inclusion rectangle contains: about 0.7 square kilometers (real data) 2) Area available to the empire builders: 300,000 sq km (real data) 3) Area of the appropriate building area: 30,000 sq km (conservative assumption) Therefore the probability that the builders chose randomly just those square kilometers of land to build the pyramid complex, is equal to:
For Teotihuacan, official archeology attributed the building of the pyramids to the people of the Toltecs, but later abandoned this hypothesis to include other non-defined people.
So, given the uncertainty about the date of construction and the people who built it, we can speculate that the builders had available a vast territory, probably at least 100,000 times the size of the pyramid complex. It is a very conservative assumption, given the complexity, the majesty and the splendor of Teotihuacan, it would in fact be reasonable to think that the builders of an empire had at their disposal a much larger area (as a comparison just think about the size of the Egyptian empire at the time the construction of the pyramids of the Giza plateau, which is more than double this). To simplify the calculations, we have: 1) The area of the inclusion rectangle consisting of: about 1.15 sq km (real data) 2) Extent of area available to the empire builders: 115,000 sq km (conservative assumption) 3) Extent of the appropriate building area: 11,500 sq km (conservative assumption) Therefore the probability that the builders randomly chose precisely that point of the area to build the pyramid complex is equal to:
For Xianyang, the data currently in our possession are really scanty (because of military prohibitions of the Chinese government), but so as not to interrupt the study, we make a comparison with the Teotihuacan area of the inclusion rectangle and assumptions (expecting to be able at least to define the inclusion rectangle with more precision and perhaps even the extent of the empire builders’ territory): 1) The area of the inclusion rectangle: about 1.15 sq km ( chosen by analogy with Teotihuacán) 2) Extent of area available to the empire builders: 115,000 sq km (conservative assumption) 3) Extent of the appropriate building area : 11,500 sq km (a conservative assumption)
Therefore, the probability of coincidence is:
That is, the probability that the Egyptians, Pre-Hispanic and Chinese at 3 different times, in 3 different continents, have by chance built the 3 pyramids of Teotihuacán complex, Giza and Xianyang and aligned them along that particular planetary line, is approximately 2 per 100,000. 000.000 (ie "two in a hundred billion"). In commenting on this result, we have to give consideration (observation 1 bis) to the fact that any two sites on the planet Earth will always be aligned on a planetary line. So the real coincidence arises from the third site that is also built on the planetary line defined by the other two sites. The calculation should be done therefore for the newer site, but, since uncertainty about the dating of Xian is so high, we can perform the calculation for the only one of the 3 sites for which we have more data available, namely Giza. Accordingly, the probability that the Egyptians chose to build the pyramid complex of Giza at that point, aligning it by chance with the other two sites of Teotihuacán pyramids, Giza and Xianyangis:
That is about 2 chances in 100,000. Let us now analyze the observation n° 2: Starting from the probability of coincidence described above, what is the probability that the 3 peoples above, at 3 different times, in 3 different continents, after aligning 3 pyramidal complexes coincidentally along lines parallel to the planetary line, have placed the pyramids according to a similar geometric pattern?
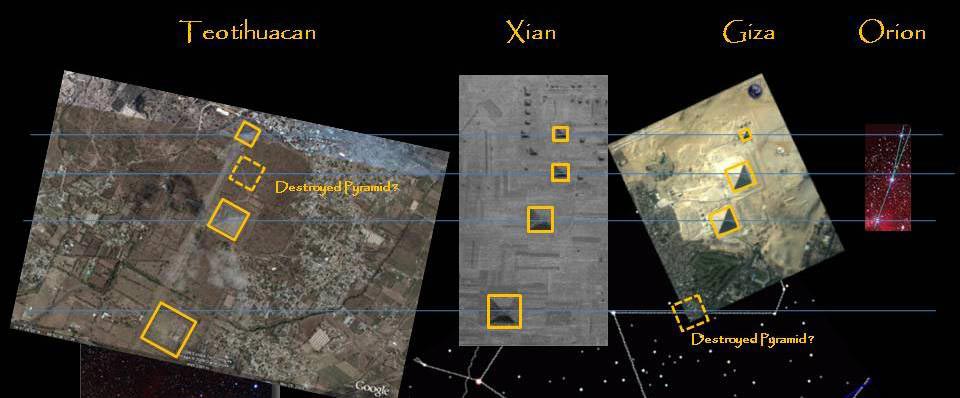
In order not to go further into the discussion, I would refer you to the studies referred to in note 1, and I would simply insert the following images to support the hypothesis of the phrase "very similar":
The images speak for themselves, there would be no need to comment on them, but it is enough to observe that for the 3 pyramid sites there is an alignment of the two major pyramids and the misalignment of the third, smallest, pyramid. The angle of misalignment between the smallest pyramid and the axis of alignment of the other two is the same (with an accuracy of a tenth of a degree) for the 3 pyramid sites. The constellation of Orion appears in the images, but this will not be considered in this study of the probability of coincidence. Also, we must establish a criterion for determining one sample area. One possible criterion is to divide up the rectangle to a grid with squares of the area of one square hectometer (a reasonable value considering the size of the base of each of the pyramids). Therefore we have at Giza 70 squares within which manufacturers could place their pyramids (by definition a grid square contains a square pyramid where the top of the pyramid coincides with the centroid of the square). The chance of the first pyramid being built just at the right position in the grid, is equal to:
The chance of the second pyramid being built just at the right position in the grid, is equal to:
The chance of the third pyramid being built just at the right position in the grid, is equal to:
Therefore, the total probability of the Pyramids having been coincidentally built just according to the current pattern is:
That is, there are 3 chances in a million.
Repeating the calculations for Teotihuacán and Xianyang, we get:
The probability for the partial observation 2 is: 0,00000000000000014%
(ie about one chance in a thousand million billion) The total probability that observation 2 and the observation 1 occur simultaneously is the following:
(ie about 3 chances out of a hundred billion billion billion) To understand this number, let us take a more every day example: - the probability of throwing a 6-sided die and getting the number 6 is equal to 1/6 or 16.66%; - by analogy, the
While the total probability for observation 2 and observation 1a to occur at the same time is as follows:
(ie about 3 chance in a thousand billion billion) So in this case, using the example of the 6-sided die, we should throw it for 29 times in succession and always obtain 6!
The It is my duty to highlight the fact that I did not take into account image 2: In fact, if I had to analyze the probability that the individual pyramids on a site are aligned with the corresponding sites of the other two, then I think I would have had difficulty even to pronounce the number that represents the total probability of coincidence. A statistician, or a skilled student of archeology, will raise a number of objections to the assumptions that I had to make to get a value for the probability of coincidence. As with my previous 2 studies (https://unina.academia.edu/SimoneScottoDiCarlo ), I emphasize that my goal is to show the order of magnitude of the problem rather than give a precise solution. Therefore, by varying the input data and varying the assumptions, you will always get such small values for the probability of coincidence as to lead one to think that the official archaeological theory has to be reviewed. Today, it is untenable to say lightly that the foregoing is only a coincidence. These are the numbers that put in mathematical form what logic and intuition have suggested for years: that there has been a deliberate choice made by builders in Teotihuacán, Giza and Xianyang to align 3 pyramid sites along planetary lines and this has not happened by chance and accordingly the pyramids also have similar geometric form. To deny this truth today means to argue that the Earth is still the center of the Universe and the Sun and the stars revolve around it. But if you accept this truth, the next step is to find out who chose to build the pyramid complex in 3 different continents in that particular way, and when. This is a huge challenge, the complexity of which requires the research effort of all the official studies and scholarship of all the enthusiasts working together, to understand and discover, without clinging to the "archaeological dogma" which is obscuring one of the most beautiful and oldest pages in human history.
|

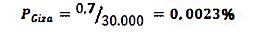


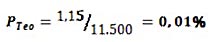
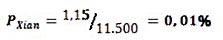
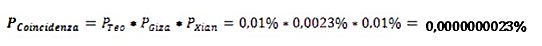
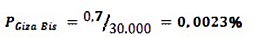
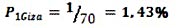

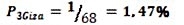
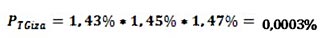
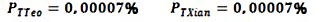
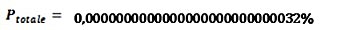
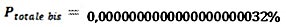

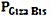 is equivalent to throwing the die 6 times in a row and always getting 6.
is equivalent to throwing the die 6 times in a row and always getting 6. 














